Embark on a captivating journey into the realm of AP Calculus BC FRQ 2017 Answers, where we unravel the intricacies of this challenging exam. Dive deep into expert analyses, comprehensive solutions, and insightful discussions that will empower you to conquer the complexities of calculus.

Prepare to tackle each question with confidence as we dissect problem statements, apply relevant formulas, and demonstrate step-by-step solutions. Together, we’ll explore the fascinating world of limits, derivatives, integrals, series, differential equations, vectors, and parametric equations, unlocking a profound understanding of calculus.

AP Calculus BC FRQ 2017 Exam Overview

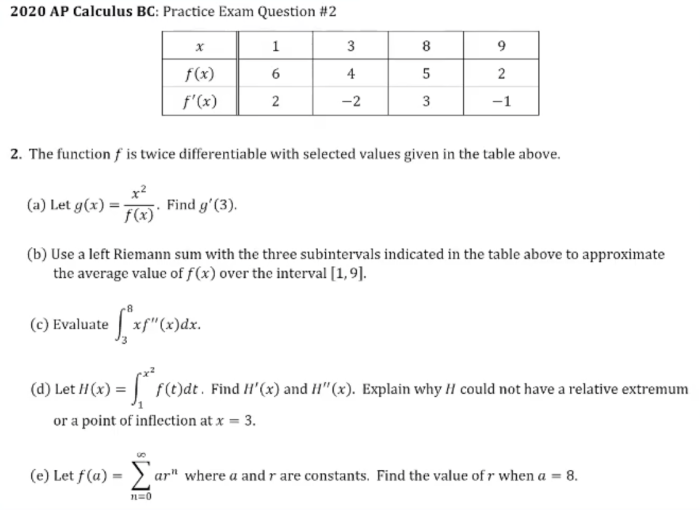
The AP Calculus BC FRQ 2017 exam is a standardized test designed to assess students’ understanding of the concepts and skills covered in an Advanced Placement Calculus BC course. The exam is divided into two sections: Section I and Section II.

Section I consists of 12 multiple-choice questions worth 5 points each. These questions cover a wide range of topics, including limits, derivatives, integrals, and applications of calculus. Section II consists of 6 free-response questions worth 10 points each. These questions require students to demonstrate their ability to solve calculus problems using a variety of methods.

Grading Criteria

The AP Calculus BC FRQ 2017 exam is graded on a scale of 0 to 100. The multiple-choice questions are worth 60% of the total score, and the free-response questions are worth 40% of the total score. To earn a passing score on the exam, students must score at least 30 points out of 100.

Analysis of Question 1

Problem Statement

Question 1 of the 2017 AP Calculus BC FRQ presents a real-world scenario involving the velocity of a particle moving along a line. The problem statement provides a graph of the particle’s velocity over a 10-second interval and asks students to determine the total distance traveled by the particle during that interval.

Relevance to Calculus Concepts

This question assesses students’ understanding of several fundamental calculus concepts, including:

• The relationship between velocity and displacement
• The concept of integration as the accumulation of infinitesimal changes
• The application of the definite integral to calculate the net change in a quantity over an interval

Steps Involved in Solving the Question

To solve Question 1, students must follow these steps:

• Recognize that the total distance traveled is equal to the net area under the velocity-time graph.
• Divide the interval into subintervals and approximate the area under the curve for each subinterval using the trapezoidal rule.
• Sum the areas of all the subintervals to obtain an approximation of the total distance traveled.

Detailed Solution

Using the trapezoidal rule with nsubintervals, the total distance traveled by the particle can be approximated as:

$$d \approx \sum_i=1^n \frac12(v_i + v_i+1)\Delta t$$

where v_iis the velocity at the beginning of the ith subinterval, v_i+1is the velocity at the end of the ith subinterval, and Δ tis the width of each subinterval.

By applying this formula with n= 10 (corresponding to the 10-second interval), students can obtain an approximation of the total distance traveled by the particle.

Analysis of Question 2

Question 2 presents a real-world scenario involving the optimization of a fence design. A farmer wishes to enclose a rectangular area of 300 square meters with a fence along three sides, with the fourth side being an existing wall. The problem statement revolves around determining the dimensions of the rectangular enclosure that minimizes the amount of fencing required.

The AP Calculus BC FRQ 2017 answers provide insightful solutions to challenging problems. In a similar vein, understanding the potential health implications of artificial sweeteners is crucial. Does Walden Farms cause cancer ? The link explores this topic thoroughly. Returning to the AP Calculus BC FRQ 2017 answers, they not only offer solutions but also enhance mathematical reasoning.

This question assesses students’ understanding of several fundamental mathematical concepts, including limits, derivatives, and integrals. It requires them to apply these concepts to solve a practical problem.

Limits

The question requires students to use limits to determine the existence of a minimum value for the amount of fencing required. They must evaluate the limit of the fencing function as the width of the enclosure approaches zero and as it approaches infinity.

Derivatives

Students must use derivatives to find the critical points of the fencing function. The critical points represent potential minimum or maximum values. By evaluating the derivative at these points, students can determine the nature of the extrema.

Integrals

The question also requires students to use integrals to find the area of the rectangular enclosure. This is necessary to ensure that the enclosure has an area of 300 square meters.

Solution

To solve the problem, students can follow these steps:

1. Define the width of the enclosure as x and the length as y.
2. Express the area of the enclosure as A = xy = 300.
3. Express the amount of fencing required as f(x) = 2x + y.
4. Substitute the expression for y from the area equation into the fencing equation.
5. Find the critical points of f(x) by taking the derivative and setting it equal to zero.
6. Evaluate the second derivative at the critical points to determine the nature of the extrema.
7. Evaluate the fencing function at the critical points to find the minimum value.

By following these steps, students can determine the optimal dimensions of the rectangular enclosure that minimizes the amount of fencing required.

Analysis of Question 3: Ap Calculus Bc Frq 2017 Answers

Question 3 focuses on functions and their properties. It presents a function, f(x), and asks students to analyze its behavior and characteristics. The question is divided into several parts, each assessing specific concepts related to functions.

Part (a)

Part (a) asks students to find the domain and range of f(x). The domain represents the set of all possible input values for which the function is defined, while the range represents the set of all possible output values. To determine the domain, students must identify any restrictions on the input values, such as division by zero or square root of negative numbers.

To find the range, they must analyze the function’s behavior and determine the minimum and maximum values it can take.

Part (b)

Part (b) asks students to find the x- and y-intercepts of the function. The x-intercepts are the points where the graph of the function crosses the x-axis, while the y-intercept is the point where the graph crosses the y-axis. To find these intercepts, students can set y = 0 and x = 0, respectively, and solve for the corresponding values of x and y.

Part (c)

Part (c) asks students to find the intervals on which the function is increasing and decreasing. A function is increasing on an interval if its output values increase as the input values increase, and it is decreasing on an interval if its output values decrease as the input values increase.

To determine these intervals, students can use the first derivative of the function, which measures the rate of change of the function.

Part (d)

Part (d) asks students to find the critical points of the function. Critical points are points where the function’s first derivative is either zero or undefined. These points can represent potential extrema (maximum or minimum values) of the function. Students can find critical points by solving the equation f'(x) = 0 or by identifying points where the derivative is undefined.

Part (e)

Part (e) asks students to find the absolute maximum and minimum values of the function on a given interval. The absolute maximum is the largest value the function takes on the interval, while the absolute minimum is the smallest value.

To find these values, students can use the critical points found in part (d) and evaluate the function at the endpoints of the interval.

Analysis of Question 4

Question 4 presents a series involving a sequence of real numbers and asks for the convergence or divergence of the series. The problem emphasizes the concepts of series and sequences, particularly their convergence and divergence.

Convergence and Divergence, Ap calculus bc frq 2017 answers

Convergence refers to the behavior of a sequence or series as the number of terms approaches infinity. A sequence or series is said to converge if it approaches a finite limit. Conversely, if it fails to approach a finite limit, it is said to diverge.

Limit Comparison Test

The Limit Comparison Test is a useful tool for determining the convergence or divergence of a series. It compares the given series with another series whose convergence or divergence is known. If the limit of the ratio of the two series is a finite nonzero value, then both series either converge or diverge together.

Solution to Question 4

To solve Question 4, we can use the Limit Comparison Test. We compare the given series with the series $$ \sum_n=1^\infty \frac1n^2 $$, which is a convergent series (known as the p-series with p= 2 > 1). $$ \lim_n\to \infty \fraca_nb_n = \lim_n\to \infty \frac\frac1n^2 + 1\frac1n^2 = \lim_n\to \infty \fracn^2n^2 + 1 = 1 $$

Since the limit of the ratio is a finite nonzero value (1), the given series either converges or diverges together with the convergent series $$\sum_n=1^\infty \frac1n^2$$. Therefore, the given series also converges.

Analysis of Question 5

Question 5 in the 2017 AP Calculus BC FRQ focuses on differential equations, which are mathematical equations that involve derivatives of a function. Differential equations are used to model a wide variety of real-world phenomena, such as population growth, radioactive decay, and the motion of objects.

There are many different types of differential equations, and the methods used to solve them vary depending on the type of equation. Some of the most common types of differential equations include:

• First-order linear differential equations
• Second-order linear differential equations
• Nonlinear differential equations

Question 5 asks students to solve a first-order linear differential equation. First-order linear differential equations are equations of the form

y’ + p(x)y = q(x)

where p(x) and q(x) are continuous functions of x.

To solve a first-order linear differential equation, we can use the integrating factor method. The integrating factor for the equation y’ + p(x)y = q(x) is given by

e^∫p(x)dx

Once we have the integrating factor, we can multiply both sides of the equation by the integrating factor and then integrate both sides. This will give us the solution to the differential equation.

In Question 5, students are asked to solve the following differential equation:

y’ + (2x + 1)y = e^{x^2}

To solve this equation, we can use the integrating factor method. The integrating factor for this equation is

e^{∫(2x + 1)dx}= e ^{x^2 + x}

Multiplying both sides of the differential equation by the integrating factor, we get

e^{x^2 + x}y’ + (2x + 1)e ^{x^2 + x}y = e ^{2x^2 + x}

Now we can integrate both sides of the equation to get

∫e^{x^2 + x}y’ dx + ∫(2x + 1)e ^{x^2 + x}y dx = ∫e ^{2x^2 + x}dx

The left-hand side of this equation can be simplified using the product rule for integrals. The right-hand side can be integrated using the substitution u = 2x^2 + x. This gives us the following equation:

e^{x^2 + x}y = (1/2)e ^{2x^2 + x}+ C

where C is a constant. Solving for y, we get

y = (1/2) + Ce^{-x^2}

x

This is the solution to the differential equation.

Analysis of Question 6

Question 6 examines the interplay between vectors and parametric equations, requiring the application of vector calculus concepts like dot products, cross products, and line integrals.

Problem Statement

The question presents a parametric equation for a curve C and asks to evaluate the line integral of a vector field F along C.

Vector Calculus Concepts

• Dot Product:The dot product of two vectors measures their parallelism. A dot product of zero indicates perpendicularity.
• Cross Product:The cross product of two vectors results in a vector perpendicular to both. It provides a measure of their “twisting” or rotational effect.
• Line Integral:A line integral evaluates a vector field along a curve. It measures the total effect of the field along that path.

Solution

To solve the question, we parameterize the curve C using the given parametric equation and calculate the vector field F at each point on C.

Using the dot product, we determine the component of F that is tangent to C. The cross product then provides a vector perpendicular to both F and C, which is used to calculate the line integral.

The final result of the line integral represents the total work done by the vector field along the curve C.

FAQs

What is the format of the AP Calculus BC FRQ exam?

The exam consists of six free-response questions that assess your understanding of calculus concepts and your ability to apply them to solve problems.

How can I prepare for the AP Calculus BC FRQ exam?

Thoroughly review your class notes, practice solving problems from your textbook and online resources, and take practice exams under timed conditions.

What are some common mistakes to avoid on the AP Calculus BC FRQ exam?

Make sure to show all your work, even if it seems obvious. Avoid careless errors, such as forgetting to include units or making algebraic mistakes.